Source: Complete International Mathematics For Cambridge IGCSE - David Rayner, Jim Fenson

 1.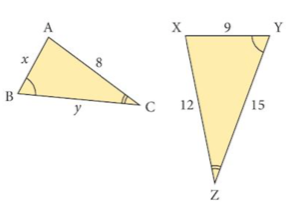
Find the sides marked with letters.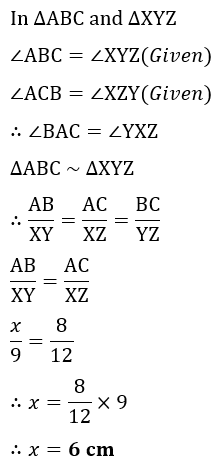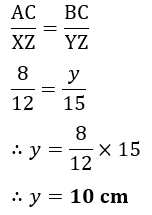
 2.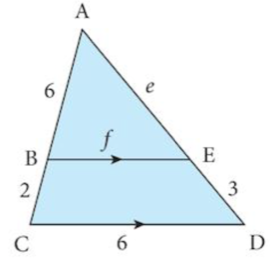
Find the sides marked with letters.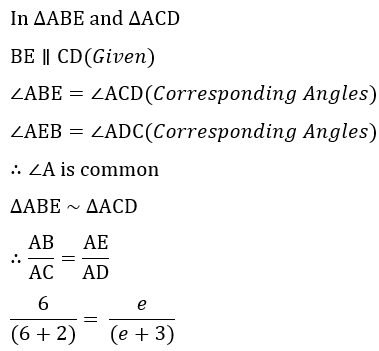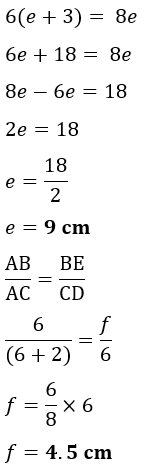
 3.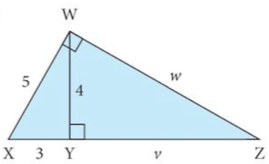
Find the sides marked with letters.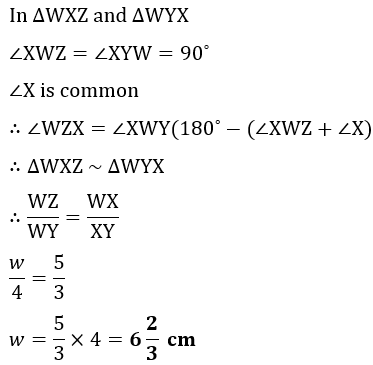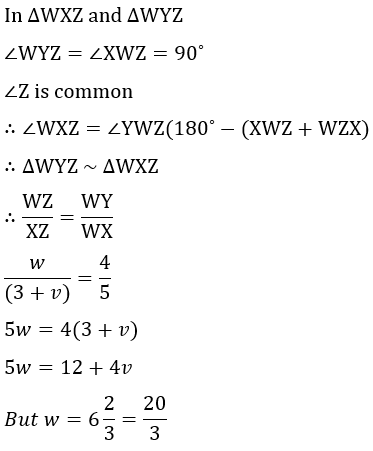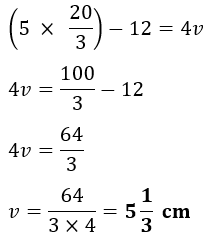
 4.
In the diagram, ∠ABE=∠ADC. 
Find the length of x.
 5.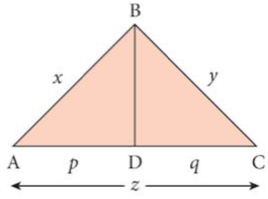
In the diagram, ∠ABC=∠ADB=90°, AD=
and DC=q. 
a) Use similar triangles to show that x² = pq.
b) Find a similar expression for y².
c) Add the expressions for x² and y²
   and hence prove Pythagoras theorem.		 a)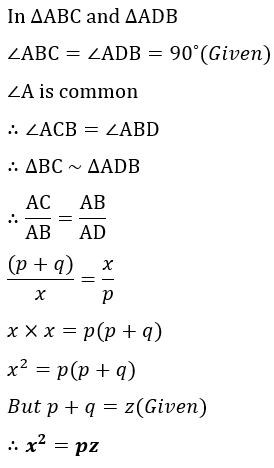 
b)

c)
 6.Triangle LMN is isosceles with LM=LN
and X and Y are point on LM, LN
respectively such that LX=LY. Prove
that triangles LMY and LNX are congruent.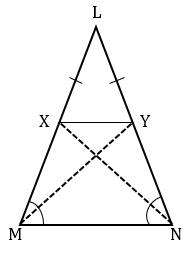
 7.Squares AMNB and AOPC are drawn on 
the sides of triangle ABC, so that they lie
outside the triangle. Prove that MC=OB.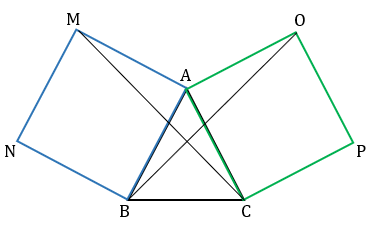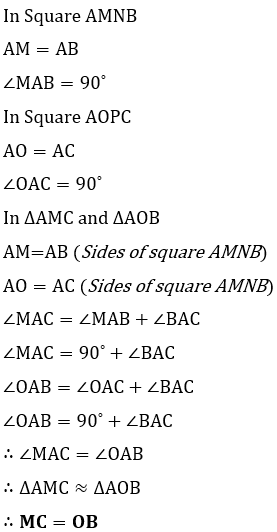
 8.In the diagram,  ∠LMN = ∠ONM = 90°.
P is the midpoint of MN, MN =2ML and 
MN = NO. Prove that :
a) the triangles MNL and NOP are congruent.
b) ∠OPN = ∠LNO
c) ∠LQO = 90°		a)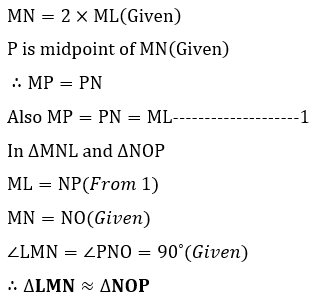

b)

c)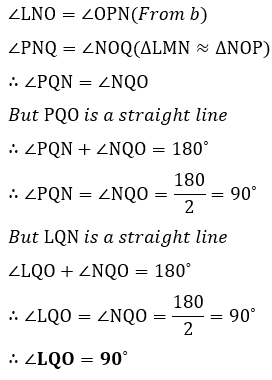

BACK TO TOP